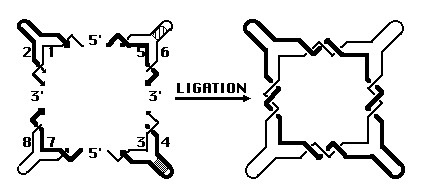The Proceedings of the 1989 NanoCon Northwest regional
nanotechnology conference, with K. Eric Drexler as Guest of Honor.
 Return to NanoCon
Top
Return to NanoCon
Top
 Return to NanoCon
Proceedings Table of Contents
Return to NanoCon
Proceedings Table of Contents
 Return to Jim's Molecular
Nanotechology Home Page
Return to Jim's Molecular
Nanotechology Home Page

NANOCON PROCEEDINGS page 5
Appendix D: Branched DNA and Nanotechnology
by Dr. Ned Seeman
Nanoscale Assembly and Manipulation of Branched DNA:
A Biological Starting Point for Nanotechnology
Nadrian C. Seeman
Department of Chemistry
New York University
New York, NY 10003
Abstract
Molecular biotechnology is a promising point from which nanotechnology can
evolve, because living systems can be thought of as a successful example
of atomic and molecular manipulation on the nano-scale. Although enzymes
manipulate atoms and molecular fragments on the Angstrom scale, biological
systems make their structural components on the nanometer scale, where weak
intermolecular energies direct the self-assembly process. This latter approach
is likely to be the simplest motif for building the first nano-scale devices.
The biological components that we are exploring for purposes of nano-scale
assembly are branched nucleic acid junctions, whose synthesis and assembly
are readily controlled with the current level of technology. It appears
possible to use these junctions to make stick-figures and crystalline networks.
These objects can be used for scaffolding to orient and juxtapose other
molecules to form biochips, nanomanipulators and multienzyme complexes.
Strategies for the synthesis of involve elimination of structural alternatives.
Potential cloning strategies for DNA structures are based on single-stranded
vectors.
Nanotechnology as an outgrowth of biotechnology
Nanotechnology may be approached from two directions, either top-down or
bottom-up. The top-down approach was originally delineated by Feynman
(1961), who suggested that machines could be constructed that could
build successively smaller machines until the nano-scale was reached. Miniaturization
of the sort Feynman suggested is actively pursued by numerous investigators
(e.g., Angell, et al., 1983; A.
Robinson, 1985). The bottom-up approach entails making objects and devices
on the nano-scale from molecular and macromolecular components. There is
good reason to believe that this approach is practical to some extent, since
living systems already exemplify its success: cells manipulate chemical
structure on the Angstrom scale via their enzymatic proteins; in addition,
they contain self-assembling structural components, also usually proteins,
although these are on the nanometer scale. Drexler
(1981) has recognized the relationship between the tasks performed by
the cell and the mechanical tasks fulfilled by our macroscopic technology.
It is important to differentiate the manipulation of intact molecules from
the manipulation of individual atoms or molecular fragments to form new
covalent molecules. Intact molecules bind to each other non-covalently by
means of weak forces: One of the best-known tight-binding interactions involves
two macromolecules in a complex, Lac repressor protein and its DNA
operator sequence. Under cellular conditions the dissociation constant of
the complex is 10-13 M (e.g., Stryer,
1988), corresponding to 18.2 Kcal/mole. Such intermolecular binding
results from a combination of numerous weak interactions, such as hydrogen
bonds, complementary van der Waals contacts and electrostatic interactions.
The total number of covalent bonds in the molecules involved in a protein-DNA
complex is over 1000. Breaking any of these covalent bonds would cost far
more energy than pulling the molecules away from each other: Energies associated
with single bonds found in biological systems are, H-O, 110, H-N, 84, H-C,
99, C-C, 80, C-N, 62, and C-O, 81 Kcal/mole (e.g., Moore,
1963). The making and breaking of covalent bonds in biological systems
is almost always catalyzed by their protein-based catalysts, the enzymes.
On the other hand, self-assembly is spontaneous. Manipulation that involves
the breaking or formation of covalent bonds requires the control of processes
in which large amounts of energy can be liberated or consumed (depending
on reference states). Although protein engineering is a burgeoning field
today, the efforts of workers in that area are largely directed at changing
the stabilities and specificities of existing enzymes, rather than catalyzing
new reactions. For example, work is in progress to change the features and
substrates of molecules such as restriction enzymes, tRNA synthetases, proteases
and reductases (e.g., Oxender and Fox, 1987),
and much is hoped from abzymes (Pollack, et al.,
1986; Tramantano, et al., 1986), but the
goal of making new ceramics with protein-based enzymes is more remote.
Specific intermolecular self-assembly is much easier to control. However,
because a large number of weak interactions are typically involved in this
process, the scale of assembly must increase from the Angstrom scale to
the nanometer scale. The following discussion is predicated on the premise
that the low energies required for assembly and manipulation in an intermolecular
context are easier to treat than the higher energies necessary to make and
break chemical bonds. Thus, it is likely that we will move enzymes around
and orient them to make new multi-functional complexes in the near future,
but a much larger time-frame will be necessary to develop catalytic activities
that do not deal with molecules of biological origin.
Because the predominant examples of nanotechnology in nature derive from
living systems, it is reasonable to look to those systems for the components
of the first nanotechnological objects and devices. Thus, nanotechnology,
which can come from many routes, is likely to evolve in part from molecular
biotechnology. Because most of the functions performed within the cell are
executed by proteins, most of the thinking has been directed towards those
molecules. Although some progress has been made in this direction (e.g.,
Regan and DeGrado, 1988; Degrado,
et al., 1989), the design and manipulation of protein structure is not
straightforward: the largest gap in our understanding of the Central Dogma
of Molecular Biology is the way in which the 3-D structure of proteins is
directed by their sequence.
Nucleic acids as components of nanotechnological
systems
For some years, we have been working on an alternative self-assembling biological
system, the nucleic acids DNA and RNA. As known to all, these polymeric
molecules can form double helical structures from two anti-parallel strands
whose sequences are complementary: an adenine (A) on one strand pairs with
a thymine (T) on the opposite strand, while the same relationship exists
between guanine (G) and cytosine (C). The inherent difficulty of imagining
DNA as an appropriate material for nano-scale construction is that the pre-eminent
feature of nucleic acid molecules is that they are organized about linear
helix axes; although the double helix may supercoil or writhe over a long
distance, the helix axis remains unbranched. From a structural point of
view, this means that nucleic acids correspond to line segments, which can
only form longer lines or circles; the nucleic acid vertex is absent from
most considerations of double helical DNA or RNA.
While attempting to model intermediates in genetic recombination that are
naturally unstable, we devised minimal-symmetry sequence-specifying algorithms
(Seeman, 1981, 1982;
Seeman and Kallenbach, 1983) for making specific
stable, self-assembling, branched nucleic acid complexes from synthetic
oligonucleotides. An example of such a 'junction' structure with four arms
is shown in Figure 1. This junction is termed
'immobile', because it contains no homologous twofold sequence symmetry
about its center; junctions with small amounts of such sequence symmetry
are called semi-mobile or partially mobile. As a practical matter, we have
concentrated on DNA, rather than RNA or RNA-DNA hybrids, primarily because
its synthesis is conveniently accomplished by solid-state automated techniques
(Caruthers, 1982) and because it is less sensitive
to hydrolysis. We and others have characterized and modeled these molecules
and their interactions extensively (Kallenbach,
et al., 1983a, 1983b; Seeman,
et al., 1985; Wemmer, et al., 1985; Kallenbach
and Seeman, 1986; Marky, et al., 1987; Cooper
and Hagerman, 1987; Churchill, et al., 1988;
Chen, et al., 1988; Duckett
et al., 1988; Mueller, et al., 1988; Petrillo,
et al., 1988; Seeman, 1988; Seeman
and Kallenbach, 1988; Seeman, et al., 1989).
For purposes of this discussion, the most important fact that has been established
about junctions is that strands designed by these algorithms can self-assemble
to form branched complexes, even though linear duplex nucleic acids are
intrinsically more stable than branched ones (Courey
and Wang, 1983). We have explored junctions with 3-6 arms in a preliminary
study, and find that those with five and six arms are less stable than those
with three and four arms (Mueller, et al., 1987).
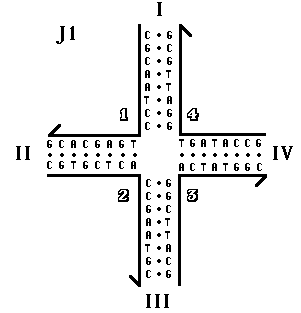
Figure 1. A Stable DNA Branched Junction.
The junction shown, called J1, is composed of four strands of DNA, labeled
with Arabic numerals. The 3' end of each strand is indicated by the half-arrows.
Each strand is paired with two other strands to form a double helical arm;
the arms are numbered with Roman numerals. The hydrogen bonded base paring
that forms the double helices is indicated by the dots between the bases.
The sequence of this junction has been optimized (Seeman
and Kallenbach, 1983) to minimize symmetry and non-Watson-Crick base
pairing. Because there is no homologous twofold sequence symmetry flanking
the central branch point, this junction cannot undergo the isomerization
reaction known as branch point migration. This junction is stable, and has
been extensively characterized (see text).
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
Construction with nucleic acid branched junctions
Genetic engineering with unbranched DNA molecules uses cohesive 'sticky'
ends on different fragments to direct their assembly in a particular order
(Cohen, et al, 1973): these single-stranded
regions will pair with complementary single-stranded regions on other molecules
to form complexes that are then annealed to covalency by DNA ligase (e.g.,
Maniatis, et al., 1982). Nucleic acid branched
junctions can be treated in the same way. Attachment of particular sticky-ends
to a branched nucleic acid structure converts it, in principle, to a highly
specific valence cluster with addressable ends (Seeman,
1981, 1982, 1985a,
1985b). These branched molecules are building
blocks that can, in principle, be assembled into stick-figures and N-connected
networks, in which the edges are made from double helical DNA, and the vertices
correspond to the branch points. Assembly of a 4-arm junction into a quadrilateral,
with the potential of forming a 2-D lattice is illustrated in Figure
2. It is worth noting that this system is inherently a three-dimensional
system, since the edges are helices, rather than parallel ladders, as they
are drawn in Figure 2.
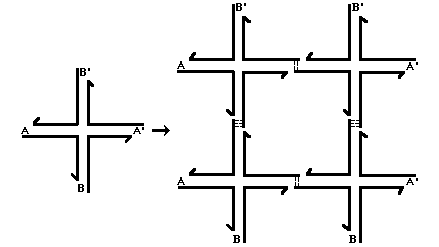
Figure 2. Formation of a two-dimensional
lattice from an immobile junction with sticky ends. A is a sticky
end and A' is its complement. The same relationship exists between B and
B'. Four of the monomeric junctions on the left are complexed in parallel
orientation to yield the structure on the right. Note that A and B are different
from each other, as indicated by the pairing in the complex. DNA ligase
can close the gaps left in the complex. Note that the complex has maintained
open valences, so that it can be extended by the addition of more monomers.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
We have demonstrated that individual three-arm junctions can be ligated
together to form 2-connected linear and macrocyclic oligomers, using a variety
of junction-flanking sequences (Ma, et al., 1986).
The same experiment has been performed with all six pairs of arms of a particular
4-arm junction (Petrillo, et al., 1988). In
all these cases, the first macrocycle formed is the trimer, while the final
products are at least as high as linear and cyclic decamers and hexamers,
respectively. The ability to form all these different closed macrocycles
indicates that junctions are flexible, both a problem and an advantage in
using them as structural components.
The ligation experiments described so far have all been performed with junctions
separated by two turns of DNA in the final product. When the same experiment
is done with one and a half turns of DNA between junctions, the ladder of
macrocyclic products begins with the tetramer, although a trace of the highly
strained trimer can be detected (Petrillo, et al.,
1988). This result demonstrates the importance of taking twist into
account when engineering with nucleic acids. Parallel line representations
of DNA, such as Figure 2, are only valid if
the junctions are separated by an integral number of turns of DNA. The geometrical
aspects of construction with helical components have been analyzed (Seeman,
1985a), and an interactive computer-graphics program has been written
to facilitate design in this context (Seeman, 1985b).
The ligation experiments described above involve repetitive linkage of a
single type of junction molecule. In addition, we have demonstrated that
assembly of a particular structure can be directed by utilizing a set of
junctions with individually addressable sticky ends (Chen,
et al., 1989). In this case, a particular quadrilateral was designed
to yield two hextuply linked circles when its four component junctions are
ligated (Figure 3). A pentameric restriction
site is incorporated into one exocyclic arm of each circle. These are particular
loci where DNA is recognized and cleaved by endonucleases called restriction
enzymes. When the DNA has been cleaved here after the synthesis, its sequence
can be determined (Maxam and Gilbert, 1977)
to establish the proper assembly of components. We have performed this analysis
to demonstrate formation of the desired product. Thus, it appears that the
addressability of the sticky ends is indeed a reliable property of this
self-assembling system (Chen, et al., 1989).
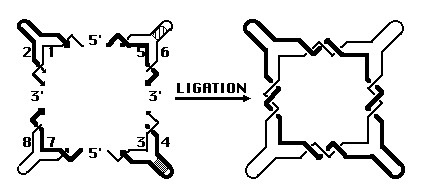
Figure 3. The Scheme of Synthesis of a DNA Quadrilateral.
The individual reactant junctions are shown on the left of the figure and
the product is shown on the right. For clarity, the double helicity of the
DNA has been represented merely as parallel lines in the vicinity of the
branch sites, and is confined to regions distal to the branch sites; nevertheless,
all the twisting expected on the main cycle is shown on both sides of the
figure. On the left, thick strands and the thin strands are associated in
pairs to form 3-arm junctions, in which one 'exocyclic' arm is closed in
a hairpin loop. Arrowheads represent the 3' ends of individual strands.
Strand numbering is indicated on the left by the numbers from 1 to 8. The
5' and 3' symbols indicate the sense of the single-stranded overhangs. The
overhangs are all on the short strands. The filled region in the exocyclic
stem formed by strand 6 represents a restriction site, while the filled
region in the stem of strand 4 corresponds to a different restriction site.
When the ligation is complete, the four junctions are expected to form two
intersecting DNA circles that are linked 6 times.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
I have described a self-assembling system for making stick-figures, in which
the ends are highly addressable. In addition, the individual edges are relatively
stiff: The persistence length of DNA is usually taken to be about 600 Å
(Allison and Schurr, 1979; Barkeley
and Zimm, 1979), although it can vary from 450 Å to 1000 Å,
depending on conditions (Hagerman, 1988); most
of the applications envisioned here entail specific links every 70-100 Å
(2-3 turns of DNA). The least favorable feature of this system is that the
'valence angle' between any pair of arms is not fixed, but may vary extensively
from junction to junction, or even within the same junction, over time.
This flexibility of the junction cluster may be partly advantageous for
junctions with four or more arms: When four or more different objects are
attached to a central object to form a rigid 3-dimensional cluster, the
cluster forms in both right-handed and left-handed mirror images of each
other. The presence of both mirror images in a reaction mixture can yield
a multitude of products, but flexible junctions are not frozen into left-handed
and right-handed configurations of sticky ends before ligation occurs.
Flexibility is not necessarily a problem in directing assembly for an isolated
device, because sticky-ended associations can be used to specify connectivity;
since no symmetry is necessary among the various sticky ends employed, flexibility
need not lead to ambiguity in the connectivity of the object. Nevertheless,
the geometry of an object is not fixed by connectivity in a flexible system.
For example, closure of a belt-like object to form a prism can yield, in
principle, two isomers, with one side of the belt or the other on the outside
of the prism. Furthermore, for an infinite device, such as a crystal (Seeman,
1981, 1982) or a crystal-based biochip (Robinson
and Seeman, 1987) the two complementary ends of adjacent unit cells
are inherently identical throughout the lattice. Therefore, flexible connections
can lead to cyclization and hetereogeneous structures, because different
angles may be formed between different repeating units. In macroscopic terms,
one might think of this system as being like Tinker Toys, in which the linear
connectors are made from moist, but not fully hydrated pasta, such as double
helical rotini, while the junction pieces that they impale are made out
of marshmallows.
In general, in order to minimize these 'valence angle flexibility' assembly
problems, one must utilize synthetic protocols and structural motifs that
minimize sensitivity to flexibility. Consider, for concreteness, the 5-connected
network shown in Figure 4. This drawing shows
the lattice of interstitial octahedra formed by a periodic array of truncated
cubes. In principle, this array could be constructed from repeat units of
individual rigid octahedra having external arms that contain the appropriate
sticky ends. However, since each individual junction is so flexible, connections
between octahedral corners on different octahedra cannot be guaranteed to
form squares; triangles or pentagons, for example could form with an octahedron
at each vertex. With this flexible system, one would need to construct a
specific square array of these octahedra, and perhaps the entire cube-like
array in Figure 4, because of the flexibility
of the joints flanking the vertices. Thus, connecting nucleic acid polyhedra
by corners or edges does not appear to guarantee the extent of control necessary
to construct pre-specified periodic lattices of these objects.
Face-connected motifs appear to be a means around this sort of problem.
For example, the assembly of the truncated octahedra of Figure
5 ought to be immune to junction flexibility, because orientation is
controlled by multiple contacts in each direction. This type of inter-object
contact ought to be fostered under stringent hybridization conditions to
avoid mispairing, which can produce improper linkages. Note that an extra
aspect of specificity can be incorporated into the truncated octahedra of
Figure 5 by using different lengths (shown)
and different polarities (not shown), as well as different sequences for
the four contacts/face connection illustrated. Nevertheless, a sequential
association protocol is advisable, in order to avoid association of the
hexagonal faces instead of the square ones.
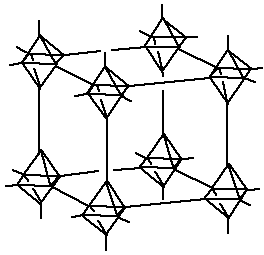
Figure 4. A 5-Connected Array of Octahedra
Flanking a Truncated Cube. Each of the edges in this figure consists
of double helical DNA, and each of the vertices corresponds to a 5-arm junction.
In principle, this network can be extended infinitely. The unique portion
of this array is the individual octahedron. By synthesizing the entire object
shown from symmetry-minimized DNA, a face-connected cohesive pattern could
be used to extend this structure infinitely.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
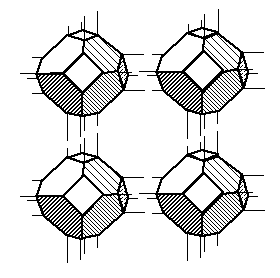
Figure 5. A Square Group of Face-Connected Truncated
Octahedra. Shown are 4 face-connected truncated octahedra, linked
at their square faces. The polyhedron formed by the connections is a cube,
drawn slightly elongated here. Only two directions of cohesion are illustrated,
but the squares on the front and back faces are available as well to form
a 3-D lattice from this basic building block. The individual lines connecting
the truncated octahedra are made from double helical DNA. The arrowheads
indicate the longer double helices in each pair that are to be ligated.
The set of these on each square face is the lock-and-key feature on which
specificity relies in this illustration. Note that the arrangement of long
and short connecting pieces is used in the horizontal direction is different
from that in vertical direction. No use is made of polarity (3' or 5' overhangs)
in this figure to increase specificity, but this feature can also be employed,
as noted in Figure 3. Extension of this array
in the direction out of the page will create a central cavity shaped like
a great rhombicuboctahedron (Williams, 1979).
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
Restriction-growth cycles
A general synthetic strategy suggested by our experience with the quadrilateral
(Chen, et al., 1989) involves successive cycles
of purifying covalently closed intermediates by enzymatic digestion, followed
by restriction. The major step in purifying the quadrilateral is treatment
of the crude ligation product with exonuclease III, which removes impurities
that contain free ends. In this synthesis, only one impurity resists exonuclease
treatment, and that substance is readily purified away from the desired
product by electrophoresis. Besides exonuclease resistance, covalently closed
intermediates are stable under denaturing conditions, while species with
free ends will denature. Thus, the formation of covalently closed intermediates
is a convenience in synthesis.
Of course, covalently closed intermediates contain no ends. Fortunately,
it is possible to create new cohesive ends by treating these intermediates
with restriction endonucleases, once the exonuclease has been removed. This
technique is shown in Figure 6, illustrating
the 2-step synthesis of a cube. One must take precautions not to increase
the symmetry of the cohesive ends unnecessarily by restriction; the sequence
symmetry of most restriction sites is known to be twofold (e.g., Yuan,
1981). This problem may be overcome in at least two ways: (1) use of
the few restriction enzymes that do not cut symmetrically, or (2) use of
multiple attachment sites for a single contact, as illustrated above in
Figure 5. The different lengths of the 4 arms
connecting faces of the truncated octahedra provide the lock-and-key on
a larger scale; so long as they are different, symmetry in the individual
cohesive ends will not destroy control of associations in the entire contact
region. This approach is analogous to the high specificity of base pairs
that derives from the placement of hydrogen bonds in a double helical context:
although A is complementary to G, for example, the backbone imposes a structure
that causes A-G pairing to cost free energy, relative to pairing with pyrimidines.
Similarly, forming one connection between polyhedra, rather than four, costs
free energy at stringent temperatures, and will not be favored.
Figure 6.
Ligation-Restriction-Ligation Cyclic Scheme for the Synthesis of a Cube
in two steps.
Step 1 -- Combine "Squares"
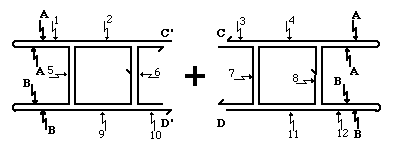
to Form a "Ladder"
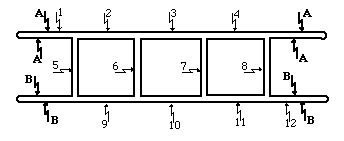
Step 2 -- Activate the Ends

ligate, and the cube is complete
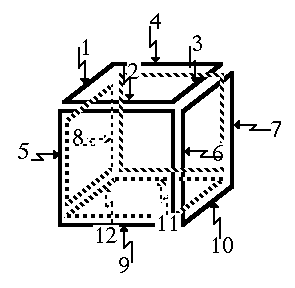
Figure 6. Ligation-Restriction-Ligation
Cyclic Scheme for the Synthesis of a Cube. Half-arrows indicate
the 3' ends of strands. Bold letters are symbolic of cohesive ends; complements
are indicated by primes. Note that A and B cohesive ends are masked by hairpins.
The numbered thunderbolt arrows are sites of restriction endonuclease sites
needed for analysis of product; each edge contains a unique restriction
site. Every edge is designed to be two helical turns long, and thus the
parallel-line representation of double helix between junctions is valid.
The first step of synthesis involves hybridization of two unique 3-strand
squares, whose corners are 3-arm junctions. All 5' ends are phosphorylated
and available for ligation at this stage. The ligation reaction seals the
central strands of the squares and joins C and C', as well as D and D'.
When the ligation is complete, the 3-square, belt-like covalently-closed
intermediate is formed in molecules we wish to use further. All contaminating
species containing free ends are digested with exonuclease III at this stage.
A and B sites are then exposed by restriction, thereby providing new sticky
ends for further growth. The 5' ends in A, A', B and B' are then phosphorylated,
and the second ligation joins A and A', B and B' to yield closure of the
cube.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
DNA networks as molecular scaffolding
It is clearly desirable to be able to orient a molecule relative to any
other molecule. This capability could provide fundamental information about
the structural and thermodynamic parameters that characterize the relationship
between the molecules, as discussed below in the section on nanomanipulation.
In addition, there are technological reasons for wishing to achieve such
ends. DNA is particularly well-suited for use as a scaffolding medium, since
it is a thick (2 nanometer diameter), stiff molecule over a range of a few
nanometers, a molecule whose structure is unlikely to be perturbed markedly
by attaching smaller non-interactive molecules to it. Neutral versions of
DNA can be made (Froehler, 1986) which may prove
more useful than the naturally-occurring polyanion.
We have recently discussed the use of DNA as a scaffold for the assembly
of an information storage device or biochip, consisting of a network of
organic conductors, molecular switches and an oxidation-reduction-based
metallic bit (Robinson and Seeman, 1987): The
forms of attachment suggested include both covalent links between the DNA
and the conducting network, as well as non-covalent links that exploit the
specificity with which both proteins and drugs recognize specific sites
on DNA molecules. Of course, other nucleic acid molecules can also be used
for attachment by forming non-covalent triple-stranded complexes (Moser
and Dervan, 1987); cycles formed from combinations of 'third strands'
and the assembled networks of conductors could result in topological bonding
to the DNA network, although no covalent bonds are formed with it. It is
critical that the network of other material being assembled by the DNA scaffolding
be capable of being assembled in parts. In order to achieve this modular
feature, we suggested making 'junctions' in the conducting material based
on coordination complexes (Robinson and Seeman,
1987).
The biochip we proposed is predicated on the ability to self-assemble a
3-dimensional crystal of branched DNA networks, to which the conducting
material and active components are appended. Addressability of individual
unit cells within the crystal is based on the ability to address a given
column within the crystal from each direction: When switches triggered in
two perpendicular directions are both open, then information may be placed
into or retrieved from a given unit cell via the third direction.
Scanning-tunneling microscopes (e.g., Beebe, et
al., 1989) and atomic force microscopes (Drake,
et al., 1989) already have 2-dimensional addressing capabilities much
finer than needed for forming the interfaces to macroscopic circuitry. The
device is designed to work at electronic speeds, rather than phonon speeds,
to which molecular mechanical devices are limited. The dimensions of each
bit in the device are 280 by 280 by 374 Å; an array of these elements
1 millimeter on a side (smaller than typical diamonds in a ring) would contain
3.4 x 1013 bits, enough to naively encrypt each of the approximately
107 books ever written three times apiece. The reader is referred
to Robinson and Seeman (1987) for a fuller explanation
of this device.
In addition to the biochip, we have suggested that stick-figures fashioned
from DNA branched junctions can function to orient and cage active biological
macromolecules (Chen, et al., 1989); the goals
in this application include the development of new multi-functional enzymes,
as well as drug delivery of therapeutic macromolecules. The large size and
expected imperturbability of DNA structure make it a good medium for the
attachment of macromolecules. Linear duplex DNA has the disadvantage that
many of the desirable distances between macromolecules are 'hidden' from
one another by being on opposite sides of the double helix. By contrast,
branched structures offer surfaces with many unoccluded loci in proximity
to each other.
The original goal of linking junctions together involved the formation of
crystals of DNA networks for use in diffraction methods (Seeman,
1981, 1982, 1985a).
All spatially periodic arrays of matter (crystals) diffract x-rays and neutrons,
and hence it is possible to determine their 3-dimensional structures in
detail (e.g., Glusker and Trueblood, 1985).
A common technique in the determination of structures involves having a
large structure host a smaller structure that binds to it in a specific
fashion (e.g., Blundell and Johnson, 1976).
Crystals of DNA networks should be able to behave as macromolecular zeolites,
in which guest macromolecules can be oriented and caged.
Nanomanipulation
Mechanical control of objects on the nanometer scale is a major concomitant
of many nanotechnological proposals. Although their motions are limited
to phonon speeds, nano-mechanical objects are of potentially great utility,
as Feynman (1961) and Drexler
(1981) have both suggested. Mechanical systems involving DNA can be
designed which use known conformational isomerizations of the molecule.
The isomerizations most readily controlled are those that involve the unwinding
of DNA. The most precipitous change in the winding of DNA is the B-->Z
transition (e.g., Rich, et al., 1984). This
isomerization involves a reversal of the handedness of the DNA double helix
from right-handed to left-handed at particular sites (primarily at alternating
pyrimidine-purine sequences). This alteration corresponds to a change in
the average winding/residue of about -65° in the affected region, from
from roughly +34.5° (Wang, 1979; Rhodes
and Klug, 1980) to -30°. A markedly less precipitous unwinding
of the DNA structure can be effected by binding particular protein molecules,
such as Lac repressor (Wang, et al., 1974),
which unwinds the DNA by roughly 45° over a stretch of about 17 nucleotide
pairs, about -2.5°/residue.
A second mechanical manipulation of DNA involving unwinding takes place
at the branch points of junctions with homologous twofold sequence symmetry;
this phenomenon is known as branch migration (Thompson,
et al., 1976). Cruciform structures in DNA are known to form in response
to underwinding closed circular DNA (Gellert, et
al, 1978; Lilley, 1980). The migratory position
of a partially mobile junction (Seeman, 1981,
1982; Chen, et al.,
1988) ought to be as readily controlled by torque exerted on opposite
arms of the junction, since its extrusive movement will relax the torque.
An example of a crystalline nanomanipulator is illustrated in Figure
7. A two-dimensional version of a single unit cell is illustrated. The
purpose of this device is to derive structural and thermodynamic information
for the same reaction. In Figure 7A, the two cubes, representing protein
molecules, for example, are in contact. Note that the cube on the right
is eccentrically attached to a piece of left-handed Z-DNA. This is the only
sequence present readily capable of achieving the Z structure. In Figure
7b, the conditions favoring Z-formation have been relaxed, and the DNA supporting
the cube on the right returns to the right-handed B conformation. This removes
the cube on the right from contact with the cube on the left, and turns
it around, as indicated by the shading. The thermodynamics of this reaction,
with appropriate baseline controls, can be measured in the same way as any
other reaction. However, given the crystalline context, structure can also
be determined by the X-ray diffraction experiment, before and after the
transition. Clearly the Z-->B driving force must be sufficient to overcome
the binding between the 'cubes' or the reaction will not occur, and nothing
will be visible crystallographically. The device shown in Figure 7 is a
2-state nanomanipulator; nanomanipulators involving more available states
are easily imagined, based on a combination of the elements described above.
Figure 7 - A crystalline nanomanipulator:
Figure 7A

a Z to B transition gives:
Figure 7B
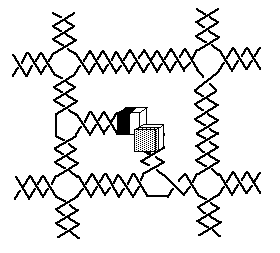
Figure 7. A Crystalline Nanomanipulator
Based on the B-Z Transition. Both portions of this figure show
the repeat unit of a two dimensional lattice of DNA. The two cubes represent
globular macromolecules, such as proteins or tRNA. One side of each cube
is shaded, to indicate polarity. All of the DNA in the figure is right-handed
B-DNA, except for the part attached to the right cube in (A), which is left-handed
Z-DNA. (A) The cube on the right is eccentrically attached to the piece
of Z-DNA, and it will move away from the cube on the left if the DNA to
which it is attached undergoes a Z-->B transition. This is shown in (B),
in which the DNA attached to the right cube has undergone a Z-->B transition.
The cube has rotated so that its opposite face is visible, and it is no
longer in contact with the cube on the left. Note that the crystalline nature
of this nanomanipulator permits both thermodynamics and structure to be
measured for the same reaction.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
Cloning
One of the obvious questions that must be asked of any DNA-based material
is, 'Can it can be cloned?' Cloning permits relatively inexpensive production
of large quantities of a substance, by having micro-organisms do the synthesis.
The answer to the question is not an unambiguous yes or no. From experience,
it is clear that chemical synthesis of DNA primary structure is much easier
than enzymatic synthesis of branched DNA secondary structure. It is obvious
that any sequence of DNA can be inserted into a vector. However, it is also
clear that if the opposite arms of a single junction, such as that shown
in Figure 1, were inserted into a plasmid, a
single round of replication would eliminate its branched character. On the
other hand, cloning into a single-stranded vector, such as M13 (Maniatis,
et al., 1982), affords opportunities not present in double-stranded
vectors. The crucial assumption on which this strategy rests is that desired
hybridization will occur, once the designed sequence is freed from the vector.
To exploit this approach, it is necessary to construct a figure whose features
can be fashioned from a continuous strand. While this may appear impossible,
the goal becomes feasible if one allows restriction of hairpin loops and
extra junction arms to create the ends of some double helical arms. Figure
8 illustrates a tetragonal pyramid whose features are formed from a
single strand. Each of the edges formed from an odd number of half-turns
of DNA are illustrated with only a single crossover, for clarity. Note that
two central edges of the pyramid are a half-turn longer than the other two,
a step necessary to maintain the continuity of the DNA.
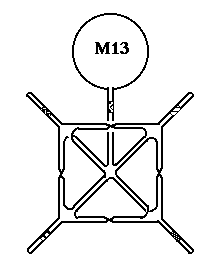
Figure 8. A Tetragonal Pyramid Formed from a
Single Long Strand and Cloned into a Single-Stranded Vector. The
pyramid is viewed from the bottom and the apex of the pyramid is in the
center of the square. The apex is a 5-arm junction, while the other four
vertices are 4-arm junctions. The apex is shown attached to the cloning
vector, single-stranded bacteriophage M13. Edges with an even number of
half-turns between vertices are shown as parallel lines, while those with
an odd number of half-turns are shown as having a single crossover. The
filled regions are restriction sites that can be cut all at once or individually
to yield the final structure. Note that any restriction site can be annealed
by ligating a hairpin over it at some future time, as desired. The rules
for constructing such an object are that strands must enter and leave a
vertex on adjacent edges of the figure, and that polarity must be maintained.
Adhering to these rules results in the edges that approach the apex of the
pyramid being mixed, two with crossovers, and two without. Thus, the pyramid
is somewhat distorted.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
If one is willing to have an external arm associated with every polygon
of a polyhedron, then the entire structure can be cloned. This is quite
general for polyhedra whose vertices are separated by an integral number
of turns of DNA, since each polygon is formed from a DNA cycle, and they
can in theory be hooked together in an arbitrary fashion. Figure
9 illustrates the Schlegel diagram of a dodecahedron to be constructed
in this fashion. The Schlegel diagram of a polyhedron is a way of representing
it in a plane: The central polygon is closest to the viewer and the polygon
on the outside of the figure is at the rear of the figure; the connectivity
of vertices is shown properly, but relative edge lengths and angles are
distorted. Twelve vertices of the dodecahedron (selected by excluding a
set of eight that lie on the body diagonals of a cube) have been used to
allow entry and exit from each of the twelve polyhedra. The connections
between polygons are indicated in Figure 9 by
the dotted lines. Once formed, the external arms can be restricted to form
the polyhedron cleanly. Additional hairpin arms can be added to this dodecahedron,
as shown in Figure 10, so that each vertex has
an external attachment point after restriction. Note that for polyhedra
with more faces than vertices, such as the icosahedron or octahedron, some
junctions must provide two extracyclic restrictable arms, rather than one
or none, as seen here with the dodecahedron.
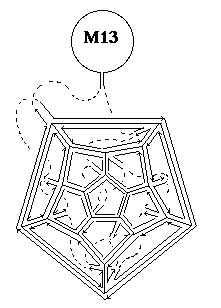
Figure 9. A Single-Stranded Representation of
a Pentagonal Dodecahedron Cloned into a Single-Stranded Vector.
A Schlegel diagram of a dodecahedron is shown in the thickest lines. Flanking
these lines are short anti-parallel arrows, drawn less heavily; these represent
the double helical DNA corresponding to each edge of the dodecahedron, with
an arrowhead indicating the 5'-->3' polarity of the strand. Each edge
is an integral number of turns long, so that the parallel line model of
DNA is valid here. Each of the twelve pentagons contains an exocyclic double
helical arm. The sites of these exocyclic segments have been selected by
eliminating the eight vertices that lie on the body diagonals of a cube.
Each exocyclic double helical segment contains a restriction site, so that
it can be severed from the connecting DNA. The dotted lines represent connecting
DNA that links the pentagons to each other and to the M13 cloning vector.
This is the DNA that will be cut away upon formation of the structure. No
attempt at topological representation is made here: all connecting DNA (dotted
lines) lies behind the polygonal DNA for purposes of clarity.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
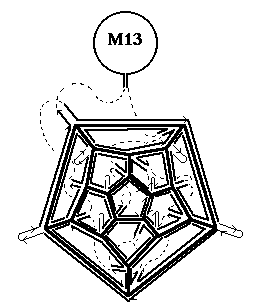
Figure 10. A Single-Stranded Cloned Pentagonal
Dodecahedron with Exocyclic Arms on All Vertices. The same conventions
apply as in Figure 9. Here the eight vertices that do not contain exocyclic
arms in Figure 9 have been provided with them in the form of hairpin loops
that contain restriction sites.
(Figure has been somewhat re-arranged from Dr. Seeman's manuscript.)
Conclusions
The most prominent example of a successful nanotechnological system is living
matter. As we understand living systems in more detail, and as molecular
biotechnology grows, it is likely that many of the earliest strands in the
fabric of nanotechnology will derive from biotechnology. Biological systems
are very facile at self-assembly on the nanometer scale, utilizing large
numbers of non-covalent interactions. It is much easier to control the low
energies necessary to direct weak intermolecular interactions, rather than
the large energies involved in making and breaking covalent bonds. Thus,
nanometer-scale construction, emulating self-assembling biological systems,
appears to be the easiest link to form between molecular biotechnology and
nanotechnology.
I have exploited this approach here in presenting a self-assembling structural
system based on nucleic acids that can be used for engineering on the nanometer
scale. The pre-eminent feature of nucleic acids that makes them so useful
for these purposes is the control one can exercise over their intermolecular
associations: the entire biotechnology industry is based on it. The introduction
of stable, branched DNA (Seeman, 1981, 1982;
Kallenbach, et al., 1983a) makes nucleic acids
a system in which this structural control may be used to make specific individual
stick-figures and N-connected networks in 3 dimensions. These materials
may in turn be useful as nanomanipulators, as well as scaffolding for other
molecules in both biochips, multienzyme complexes, diffraction studies and
biological applications.
ACKNOWLEDGEMENTS: This research has been supported by grant GM-29554
from the National Institutes of Health. Many of the ideas mentioned here
have grown out of discussions with Neville Kallenbach, whose criticisms
have vastly improved this manuscript, and with Bruce Robinson.
References
Allison, S.A. and Schurr, J.M. (1979), Chem.
Phys 41, 35-46.
Angell, J.B., Terry, S.C. and Barth, P.W (1983).,
Scientific American 248 (4), 44-55 and references therein.
Barkeley, M.D. and Zimm, B.H. (1979), J. Chem.
Phys. 70, 2991-3007.
Beebe, T.P., Jr., Wilson, T.E., Olgletree, D.F.,
Katz, J.E., Balhorn, R., Salmeron, M.B. and Siekhaus, W.J. (1989), Science
243, 370-372.
Blundell, T.L., and Johnson, L.N. (1976), Protein
Crystallography, Academic Press, New York, pp. 404-419.
Caruthers, M.H. (1982), in Chemical and Enzymatic
Synthesis of Gene Fragments, ed. by H.G. Gassen and A. Lang, Verlag Chemie:
Weinheim, 71-79.
Chen, J.-H., Churchill, M.E.A., Tullius, T.D.,
Kallenbach, N.R. and Seeman, N.C. (1988), Biochemistry 85, 6032-6038.
Chen, J.-H., Kallenbach, N.R. and Seeman, N.C.
(1989), J. Am. Chem. Soc. in press.
Churchill, M.E.A., Tullius, T.D., Kallenbach,
N.R. and Seeman, N.C. (1988), Proc. Nat. Acad. Sci. (USA) 85, 4653-4656.
Cohen, S.N., Chang, A.C.Y., Boyer, H.W. and Helling,
R.B. (1973), Proc. Nat. Acad. Sci. (USA) 70, 3240-3244.
Cooper, J.P. and Hagerman, P.J. (1987), J. Mol.
Biol. 198, 711-719.
Courey, A.J. and Wang, J.C. (1983), Cell 33,
817-829.
Degrado, W.F., Wasserman, Z.R. and Lear, J.D.
(1989), Science 243, 622-628.
Drake, B., Prater, C.B., Weisenhorn, A.L., Gould,
S.A., Albrecht, T.R., Quate, C.F., Cannell, D.S., Hansma, H.G. and Hansma,
P.K. (1989), Science 243, 1586-1589.
Duckett, D.R., Murchie, A.I.H., Diekmann, S.,
van Kitzing, E., Kemper, B. and Lilley, D.M.J. (1988), Cell 55, 79-89.
Drexler, K.E. (1981), Proc. Nat. Acad. Sci. (USA)
78, 5275-5278. [Note: Drexler's paper "Molecular
engineering: An approach to the development of general capabilities for
molecular manipulation" is now available on the Web.]
Feynman, R.P., in Miniaturization, ed. by H.D.
Gilbert, Reinhold Publishing Corp., New York, 282-296 (1961). [Note: Feynman's
paper "There's
Plenty of Room at the Bottom" is now available on the Web.]
Froehler, B.C. (1986), Tett. Lett. 27, 5575-5578.
Gellert, M., Mizuuchi, K., O'Dea, M.H., Ohmori,
H. and Tomizawa, J (1978), Cold Spring Harbor Symp. Quant. Biol. 43, 35-40.
Glusker, J.P. and Trueblood, K.N. (1985), Crystal
Structure Analysis, 2nd ed., Oxford University Press, New York.
Hagerman, P.J. (1988), Ann. Rev. Biophys. &
Biophys. Chem 17, 265-286.
Kallenbach, N.R., Ma, R.-I. and Seeman, N.C.
(1983a), Nature (London) 305, 829-831.
Kallenbach, N.R., Ma, R.-I., Wand, A.J., Veeneman,
G.H., van Boom, J.H., and Seeman, N.C. (1983b), J. Biomol. Str. and Dyns.,
1, 158-168.
Kallenbach, N.R. and Seeman, N.C. (1986), Cmts.
on Cell. and Mol. Biophys. 4, 1-16.
Lilley, D.M.J. (1980), Proc. Nat. Acad. Sci.
(U.S.A.) 77, 6468-6472.
Ma, R.-I., Kallenbach, N.R., Sheardy, R.D., Petrillo,
M.L. and Seeman, N.C. (1986), Nucl. Acids Res. 14, 9745-9753.
Maniatis, T., Fritsch, E.F. and Sambrook, J.,
Molecular Cloning, Cold Spring Harbor Laboratory (1982).
Marky, L.A., Kallenbach, N.R., McDonough, K.A.,
Seeman, N.C. and Breslauer, K.J. (1987), Biopolymers 26, 1621-1634.
Maxam, A.M. and Gilbert, W. (1977) Proc. Nat.
Acad. Sci. (U.S.A.) 74, 560-564.
Moore, W.J. (1963), Physical Chemistry, Prentice-Hall,
Englewood Cliffs, NJ, p. 59.
Moser, H.E. and Dervan, P.B. (1987), Science 238,
645-649.
Mueller, J.E., Kallenbach, N.R. and Seeman, N.C.
(1987), abst. 5th Conv. Disc. Biomol. Stereodyns., 127.
Mueller, J.E., Kemper, B., Cunningham, R.P.,
Kallenbach, N.R. and Seeman, N.C. (1988), Proc. Nat. Acad. Sci. (USA) 85,
9441-9445.
Oxender, D.L. and Fox, C.F. (1987), Protein Engineering,
Alan R. Liss, Inc., New York.
Petrillo, M.L., Newton, C.J., Cunningham, R.P.,
R.-I. Ma, Kallenbach, N.R.and Seeman, N.C. (1988), Biopolymers 27, 1337-1352.
Pollack, S.J., Jacobs, J.W. and Schultz, P.G.
(1986), Science 234, 1570-1574.
Regan, L. and DeGrado, W.F. (1988), Science 241,
976-978.
Rhodes, D. and Klug, A. (1980), Nature 286, 573-578.
Rich, A., Nordheim, A. and Wang, A.H.-J. (1984),
Ann. Rev. Biochem. 53, 791-845.
Robinson, A. L. (1985), Science 228, 39-40.
Robinson, B.H. and Seeman, N.C. (1987), Prot.
Eng. 1, 295-300.
Seeman, N.C. (1981), in Biomolecular Stereodynamics,
ed. by R.H. Sarma, Adenine Press, New York, pp. 269-277.
Seeman, N.C. (1982), J. Theor. Biol. 99, 237-247.
Seeman, N.C. and Kallenbach, N.R. (1983), Biophys.
J. 44, 201-209.
Seeman, N.C. (1985a), J. Biomol. Str. & Dyns.
3, 11-34.
Seeman, N.C. (1985b), J. Mol. Graphics 3,
34-39.
Seeman, N.C., Maestre, M.F., Ma, R.-I. and Kallenbach,
N.R., in Prog. Clin. & Biol. Res. 172A: The Molecular Basis of Cancer,
ed. by R. Rein, Alan Liss Inc., New York, 1985, pp. 99-108.
Seeman, N.C. (1988), J. Biomol. Str. & Dyns.
5, 997-1004.
Seeman, N.C. and Kallenbach, N.R. (1988), in
Molecular Structure: Chemical Reactivity and Biological Activity, ed. by
J.J. Stezowski, J.-L. Huang and M.-C. Shao, Oxford University Press, Oxford,
1988, pp. 189-194.
Seeman, N.C., Chen, J.-H. and Kallenbach, N.R.
(1989), Electrophor., in press.
Stryer, L. (1988), Biochemistry, 3rd ed., W.H.
Freeman, New York, p. 803.
Thompson, B.J., Camien, M.N., and Warner, R.
C. (1976), Proc. Nat. Acad. Sci. (USA) 73, 2299-2303.
Tramantano, A., Janda, K.D. and Lerner, R.A.
(1986), Science 234, 1566-1570.
Wang, J.C., Barkeley, M.D. and Bourgeois, S.
(1974), Nature 251, 247-249.
Wang, J.C. (1979), Proc. Nat. Acad. Sci. (USA)
76, 200-203.
Williams, R. (1979), The Geometrical Foundation
of Natural Structure, Dover, New York.
Wemmer, D.E., Wand, A.J., Seeman, N.C. and Kallenbach,
N.R. (1985), Biochemistry 24, 5745, 5749.
Yuan, R. (1981), Ann. Rev. Biochem. 50, 285-315.
In addition to this paper, Dr. Seeman has also presented
a talk to this conference.

Next page of the NanoCon Proceedings
Previous page of the NanoCon Proceedings
Return to NanoCon Proceedings Table
of Contents
Return to Jim's Molecular Nanotechology Home Page
Return to Web Site Design and Authoring
Services
 Comments and correspondence? Send email to Jim Lewis:
Comments and correspondence? Send email to Jim Lewis:
nanojbl@halcyon.com
NanoCon Proceedings © Copyright 1989, by NANOCON
This page is part of Jim's Molecular Nanotechnology Web, copyright ©1996
James B. Lewis Enterprises. All rights reserved.
Last updated 24June96.
The URL of this document is: http://www.halcyon.com/nanojbl/NanoConProc/nanocon5.html
![]() Return to NanoCon
Top
Return to NanoCon
Top![]() Return to NanoCon
Proceedings Table of Contents
Return to NanoCon
Proceedings Table of Contents![]() Return to Jim's Molecular
Nanotechology Home Page
Return to Jim's Molecular
Nanotechology Home Page